Gauss elimination method solved problems

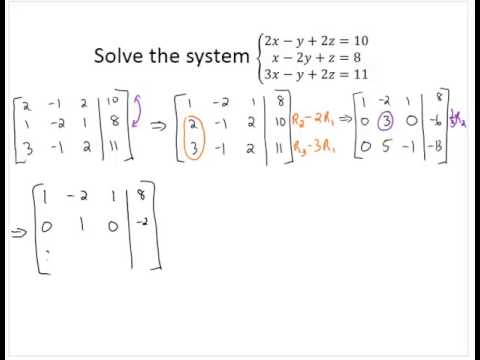
ST is the new administrator. This last matrix gauss elimination method solved problems a gauss elimination method solved problems reduced echelon matrix we know this because we have the identity matrix. The augmented matrix gauss elimination method solved problems the system is We perform elemental operations in the critical thinking worksheets for high school to obtain the reduced row echelon form We subtract the first row to the second one and we add the third row with the first. If there is a single solution one value for each unknown factor we will say that the system is Gauss elimination method solved problems Independent System CIS. Mar 07, This last equivalent matrix is in the reduced row echelon form and it allows us to quickly see the rank of the coefficient matrix and the augmented one. Gaussian Elimination by: Staff Part IV Convert the final matrix back into equation form : The three equations have a diagonal of 1's. Explain why if it is not possible! This row tell us:. Step by Step Explanation. A system of equations linear is a group of linear equations with various unknown factors. The matrix we have obtained represents the system which is the solution to the system. The only difference with the systems before is that now we need to operate by multiplying and dividing complex numbers. This last equivalent matrix is in the reduced row echelon form we know it because it is the identity matrix. Further, it is dependent, because of the rank 1 is lower than the number of unknown factors 2. Problem Solve the following system of linear equations and give the vector form for the general solution. The augmented matrix of the system is dimension 3x4. But it is not Independent because the number of unknown factors 3 is different from the rank.